SYSTEM DYNAMICS & POLE LOCATIONS:
Consider the system shown below:
Assume the system is in equilibrium and is then disturbed
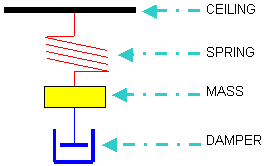
The resulting second order differential equation for the system,
representing the mass displacement from rest, to a step input has a time domain solution given by:
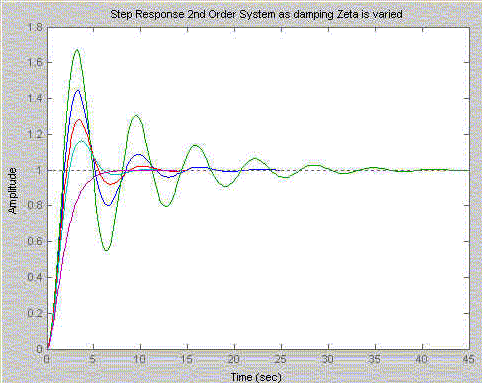
y (t) = 1 - (e--z*ω*t)*Sin( ω*√(1 - z*z)*t +Arc Cos(z) ) / √(1 - z*z)
| z = 0.1 = low damped system many oscillations in (green) output | |
| z = 1 = high damped system no oscillations in (purple) output |
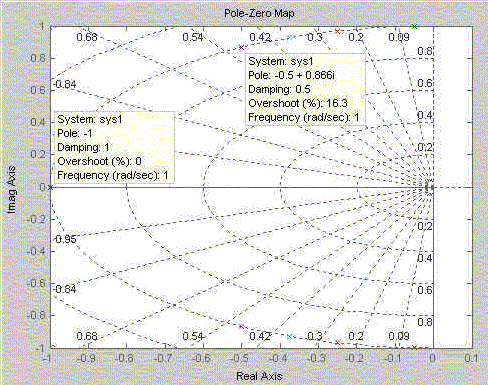
What does changing the value of z mean with respect to the pole positions of the system. As the value of damping z decreases the position of the pole approaches the imaginary axis.
| The concentric circles represent a constant frequency as damping is varied. | |
| The radial lines represent constant damping as frequency is varied |
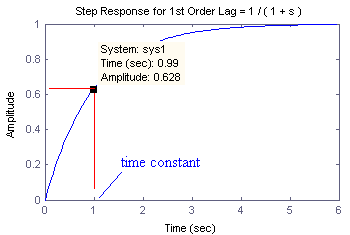
Transient Response (time domain) of systems to a step disturbance:
A first order system - the time constant of
the system is calculated by reading off the time where the output reaches 63% of
its final value.
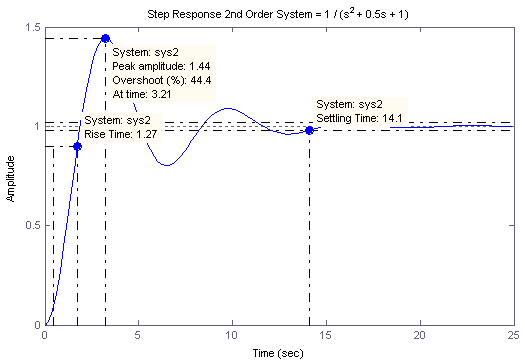
For a second order system several parameters are used to specify the response characteristics:
| Rise Time: the time difference between 10% and 90% output amplitude values | |||
Peak Amplitude: the magnitude of the maximum
overshoot seen in the response
| |||
Settling Time: the time for the output
amplitude to remain within some predefined tolerance limits
|
Calculations used for time response
characteristics:
| ωn = ωo | natural frequency | |||||||||||||||
|
Note: |
ωn = 2*π*fn | Rise time | Peak Time | |||||||||||||
| (p - b)/ωd | ||||||||||||||||
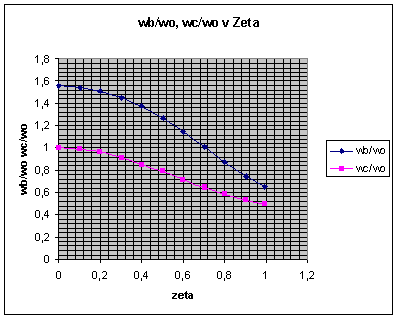
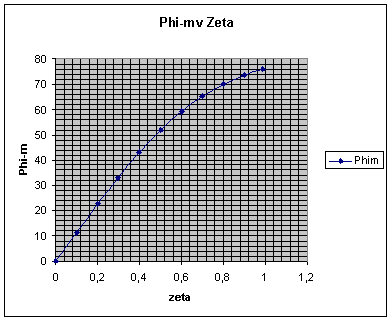
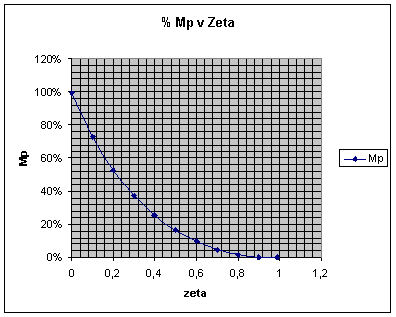
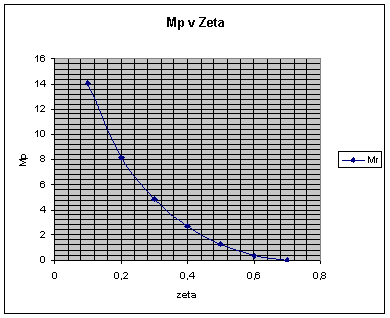
| ζ | damped frequency ωd | b | σ | Tr | Tp | Mp = M | Ts 2% | Ts 5% | Mr = Mp | ωr | ωb | ωc | ωo*Tr | ωb/ωo | ωc/ωo | Φm |
| [Hz] | arctan(ωd/σ) | ζ*ωn | [s] | [s] | % | [s] | [s] | [dB] | [Hz] | [Hz] | [Hz] | deg | ||||
| 0.001 | 1 | 1.569796327 | 0.001 | 1.5718 | 3.1416 | 100% | 4000 | 3000 | 53.9794 | 1 | 1.5538 | 1 | 1.5718 | 1.5538 | 1 | 0.11581 |
| 0.1 | 0.99499 | 1.470628906 | 0.1 | 1.67938 | 3.1574 | 73% | 40 | 30 | 14.02305 | 0.98995 | 1.5428 | 0.9901 | 1.67938 | 1.5428 | 0.9901 | 11.4217 |
| 0.2 | 0.9798 | 1.369438406 | 0.2 | 1.8087 | 3.2064 | 53% | 20 | 15 | 8.136088 | 0.95917 | 1.5096 | 0.9608 | 1.8087 | 1.5096 | 0.9608 | 22.6032 |
| 0.3 | 0.95394 | 1.266103673 | 0.3 | 1.96605 | 3.2933 | 37% | 13.333 | 10 | 4.846561 | 0.90554 | 1.4537 | 0.9144 | 1.96605 | 1.4537 | 0.9144 | 33.2733 |
| 0.4 | 0.91652 | 1.159279481 | 0.4 | 2.16288 | 3.4278 | 25% | 10 | 7.5 | 2.695407 | 0.82462 | 1.3745 | 0.8544 | 2.16288 | 1.3745 | 0.8544 | 43.1182 |
| 0.5 | 0.86603 | 1.047197551 | 0.5 | 2.4184 | 3.6276 | 16% | 8 | 6 | 1.249387 | 0.70711 | 1.272 | 0.7862 | 2.4184 | 1.272 | 0.7862 | 51.8278 |
| 0.6 | 0.8 | 0.927295218 | 0.6 | 2.76787 | 3.927 | 9% | 6.6667 | 5 | 0.354575 | 0.52915 | 1.1482 | 0.7157 | 2.76787 | 1.1482 | 0.7157 | 59.1877 |
| 0.7 | 0.71414 | 0.79539883 | 0.7 | 3.28533 | 4.3991 | 5% | 5.7143 | 4.2857 | 0.001738 | 0.14142 | 1.01 | 0.6482 | 3.28533 | 1.01 | 0.6482 | 65.1567 |
| 0.8 | 0.6 | 0.643501109 | 0.8 | 4.16349 | 5.236 | 2% | 5 | 3.75 | 0.354575 | #NUM! | 0.8709 | 0.5868 | 4.16349 | 0.8709 | 0.5868 | 69.8603 |
| 0.9 | 0.43589 | 0.451026812 | 0.9 | 6.17258 | 7.2073 | 0% | 4.4444 | 3.3333 | 2.107014 | #NUM! | 0.7461 | 0.5327 | 6.17258 | 0.7461 | 0.5327 | 73.5139 |
| 0.999 | 0.14107 | 0.141539473 | 0.99 | 21.2668 | 22.27 | 0% | 4.0404 | 3.0303 | 11.07817 | #NUM! | 0.6528 | 0.4902 | 21.2668 | 0.6528 | 0.4902 | 76.0935 |